Let:

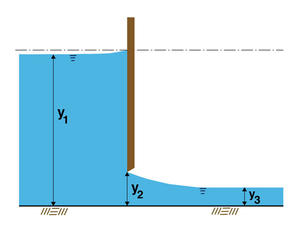
• y₁ = headwater depth, i.e., flow depth upstream of sluice gate, under parallel flow.

• y₂ = gate opening, or flow depth at sluice gate, under non-parallel flow.

• y₃ = tailwater depth, i.e., flow depth downstream of sluice gate, under parallel flow.

The conservation of energy between headwater and tailwater depths:

• y₁ + [v₁²/(2g)] = y₃ + [v₃²/(2g)]

By conservation of mass:

• q = v₁y₁ = v₃y₃

• y₁ + [q²/(2gy₁²)] = y₃ + [q²/(2gy₃²)]

• q = y₁ y₃ [2g/(y₁ + y₃)]^1/2

To express this equation in terms of the gate opening y₂, a contraction coefficient is defined:

• C_c = y₃/y₂

• q = {C_c/[1 + C_c (y₂/y₁)]^1/2} (2gy₁)^1/2 y₂

Defining a discharge coefficient:

• C_d = C_c/[1 + C_c (y₂/y₁)]^1/2

• q = C_d (2gy₁)^1/2 y₂

Defining a reference velocity:

• v₂ = C_d (2gy₁)^1/2

The unit-width discharge under a rectangular sluice gate is:

• q = v₂ y₂

The contraction coefficient C_c varies within a narrow range, between 0.598 and 0.611. A value of C_c = 0.61 has been recommended for practical use (Henderson: "Open channel flow," MacMillan, 1966).